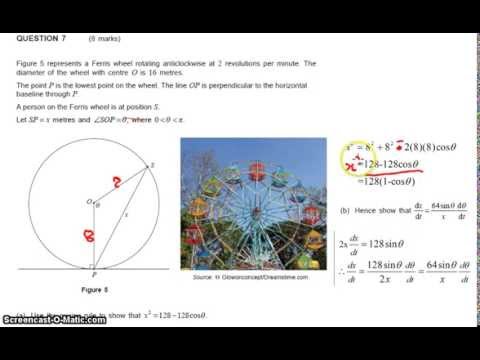
My question 7. You are given a ferris wheel rotating at two revolutions per minute and the hub has a center. Oh, and the diameter is 16 meters, so the radius is 8 meters. A person's view is at the precision such that SP is X and the angle is Theta. Using the cosine rule, it can be shown that X squared is equal to 12 + 8-1 2a cosine Theta. Now, let's examine this triangle. By applying the sine rule, we have X squared equals a squared plus X squared minus 2 times X times a times cosine Theta. However, this formula seems to have a negative sign error, so we need to rectify it in order to obtain the required result. Moving on, we can evaluate DX DT as 64 sine Theta over X. To do this, we differentiate the expressions we obtained earlier and square them. Consequently, we have 2X DX DT equals -128 sine Theta times d Theta/dt. Rearranging the expressions, we achieve the required result. Next, we move on to Part C. We have to find d Theta/dt, which represents the rate of change of the angle. By observing the rotation, we are given that the change in angle is two revolutions per minute. Since one revolution is equal to 2 pi, this implies that d Theta/dt is 4 pi. Finally, we need to find the rate at which SP is changing. We can obtain this by calculating DX DT. Using the expressions we derived from Part B and the information from Part C (d Theta/dt = 4 pi), we substitute these values and evaluate. Furthermore, in the given question, it is stated that SP is 8 meters above point P. By substituting the value of Theta into the expressions, we obtain X when X equals 28 meters, which...
Award-winning PDF software





Sace number enquiry Form: What You Should Know
Please use the following links and do not print the information from this page. We do not review the content or opinions contained in outside links. Please refer to our privacy statement and disclaimer.
online solutions help you to manage your record administration along with raise the efficiency of the workflows. Stick to the fast guide to do Form Sace Application Form, steer clear of blunders along with furnish it in a timely manner:
How to complete any Form Sace Application Form Online: - On the site with all the document, click on Begin immediately along with complete for the editor.
- Use your indications to submit established track record areas.
- Add your own info and speak to data.
- Make sure that you enter correct details and numbers throughout suitable areas.
- Very carefully confirm the content of the form as well as grammar along with punctuational.
- Navigate to Support area when you have questions or perhaps handle our assistance team.
- Place an electronic digital unique in your Form Sace Application Form by using Sign Device.
- After the form is fully gone, media Completed.
- Deliver the particular prepared document by way of electronic mail or facsimile, art print it out or perhaps reduce the gadget.
PDF editor permits you to help make changes to your Form Sace Application Form from the internet connected gadget, personalize it based on your requirements, indicator this in electronic format and also disperse differently.
Video instructions and help with filling out and completing Sace number enquiry